\begin{align}
\end{align}
Riešte rovnice s neznámou
\(t \in \mathbb{R}\):
1.
\(\sin t + \sin 2t = 0\)
-
\(\sin t + 2\sin t \cos t = 0\)
(Používame vzorce pre dvojnásobný argument.)
-
\(\sin t(1 + 2\cos t) = 0\)
(Využijeme vlastnosť, kedy sa súčin rovná nule (aspoň, keď jeden činiteľ je rovný nule.))
-
\(\sin t(1 + 2\cos t) = 0 \Leftrightarrow \sin t = 0 \vee 1 + 2\cos t = 0 \)
- Prvá možnosť:
\(\sin t = 0\)
-
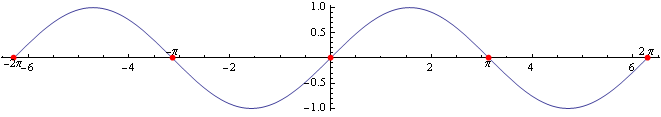
-
\(\sin t_1 = 0 \Rightarrow t_1 = 0 + k\pi, k \in \mathbb{Z}\)
(Využitím grafu a tabuľkových hodnôt funkcie sínus.)
- Druhá možnosť:
\(1 + 2\cos t = 0\)
-
\(\cos t_2 = - {1 \over 2}\)
-
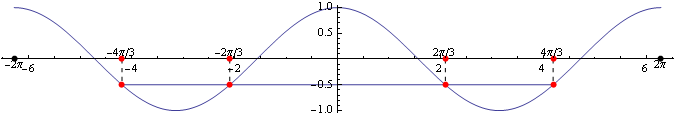
-
\(\cos t_2 = -{1 \over 2} \Rightarrow t_2 = {2 \over 3}\pi + 2k\pi; {4 \over 3}\pi + 2k\pi\)
- Výsledné riešenie napíšeme v tvare:
\(K = \bigcup\limits_{k\in\mathbb{Z}}\{\ k\pi; \frac{2}{3}{\pi}+2k\pi; \frac{4}{3}{\pi}+2k\pi \}\;.\)
2.
\(-1 = \cos 2t - \cos t\)
-
\(-1 = \cos^2 t - \sin^2 t - \cos t\)
(Používame vzorce pre dvojnásobný argument.)
-
\(-1 = \cos^2 t - (1 - \cos^2 t) - \cos t\)
-
\(2\cos^2 t - \cos t = 0\)
-
\(\cos t(2\cos t -1) = 0\)
(Využijeme vlastnosť, kedy sa súčin rovná nule (aspoň, keď jeden činiteľ je rovný nule.)
-
\(\cos t(2\cos t -1) = 0 \Leftrightarrow \cos t = 0 \vee 2\cos t - 1 = 0\)
- Prvá možnosť:
\(\cos t = 0\)
-
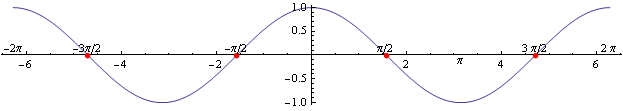
-
\(\cos t_1 = 0 \Rightarrow t_1 = {\pi \over 2} + k\pi, k \in \mathbb{Z}.\)
- Druhá možnosť:
\(\cos t_2 = {1 \over 2} \)
-
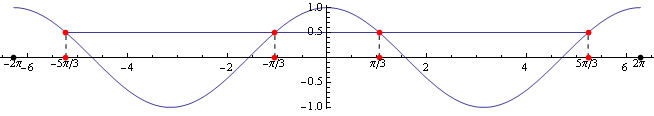
-
\(\cos t_2 = {1 \over 2} \Rightarrow t_2 = {\pi \over 3} + 2k\pi; {5 \over 3}\pi + 2k\pi \)
- Výsledné riešenie napíšeme v tvare:
\(K = \bigcup\limits_{k\in\mathbb{Z}}\{\ {\pi \over 2} + k\pi; \frac{\pi}{3} + 2k\pi; \frac{5}{3}{\pi}+2k\pi \}\;.\)
3.
\(\sin^4 t - \cos^4 t =\cos^2 2t\)
-
\((\sin^2 t)^2 - (\cos^2 t)^2 = (\cos^2 t - \sin^2 t)^2\)
(Používame vzorce pre dvojnásobný argument.)
-
\((\sin^2 t + \cos^2 t)\cdot(\sin^2 t - \cos^2 t) = (\cos^2 t - (1 - \cos^2 t))^2\)
(Použili sme vzorec
\(a^2 - b^2 = (a-b)\cdot(a+b).)\)
-
\((1-\cos^2 t + \cos^2 t)\cdot(1-\cos^2 t - \cos^2 t)=(2\cos^2 t - 1)^2\)
-
\(1-2\cos^2 t = 4\cos^4 t - 4\cos^2 t + 1\)
-
\(4\cos^4 t - 2\cos^2 t = 0\)
-
\(2\cos^2 t(2\cos^2 t - 1) = 0\)
Využijeme vlastnosť, kedy sa súčin rovná nule (aspoň, keď jeden činiteľ je rovný nule.)
-
\(2\cos^2 t(2\cos^2 t - 1) = 0\)
-
\(2\cos^2 t(2\cos^2 t - 1) = 0 \Leftrightarrow 2\cos^2 t = 0 \vee 2\cos^2 t - 1 = 0\)
- Prvá možnosť:
\(2\cos^2 t = 0\)
-
\(\cos t_1 = 0\)
-
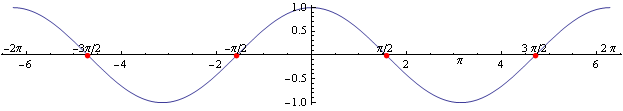
-
\(\cos t_1 = 0 \Rightarrow t_1 = {\pi \over 2} + k\pi, k \in \mathbb{Z}.\)
- Druhá možnosť:
\(2\cos^2 t -1 = 0\)
-
\(\cos^2 t_2 = {1 \over 2}\)
-
\(\cos t_2 = \pm {\sqrt{2} \over 2}\)
-
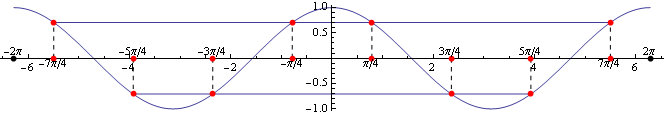
-
\(\cos t_2 = \pm {\sqrt{2} \over 2} \Rightarrow t_2 = {\pi \over 4} + k {\pi \over 2}, k \in \mathbb{Z}.\)
- Výsledné riešenie zapíšeme v tvare:
\(K = \bigcup\limits_{k\in\mathbb{Z}}\{\ {\pi \over 2} + k\pi; \frac{\pi}{4} + k{\pi \over 2} \}\;.\)
4.
\((1 + \cos 2t)\sin t = 4\cos^2 t\)
-
\((1 + \cos^2 t - \sin^2 t)\sin t = 4(1 - \sin^2 t)\)
(Používame vzorce pre dvojnásobný argument.)
- Aby výrazy v rovnici boli definované, musí platiť:
\((1 + (1 - \sin^2 t) - \sin^2 t)\sin t = 4 - 4\sin^2 t\)
-
\((2 - 2\sin^2 t)\sin t = 4 - 4\sin^2 t\)
-
\(2\sin t - 2\sin^3 t + 4\sin^2 t - 4 = 0\)
-
\(-2\sin^2 t(\sin t -2) + 2(\sin t - 2) = 0\)
-
\((\sin t - 2)(-2\sin^2 t + 2) = 0\)
Využijeme vlastnosť, kedy sa súčin rovná nule (aspoň, keď jeden činiteľ je rovný nule.)
-
\((\sin t - 2)(-2\sin^2 t + 2) = 0 \Leftrightarrow \sin t - 2 = 0 \vee -2\sin^2 t + 2 = 0\)
- Prvá možnosť:
\(\sin t - 2 = 0\)
-
\(\sin t_1 = 2 \Rightarrow t_1 \in \emptyset\)
(Také t neexistuje.)
- Druhá možnosť:
\(-2\sin^2 t + 2 = 0\)
-
\(\sin^2 t_2 = 1\)
-
\(\sin t_2 = \pm 1\)
-

-
\(\sin t_2 = \pm 1 \Rightarrow t_2 = {\pi \over 2} + k \pi, k \in \mathbb{Z}.\)
- Výsledné riešenie zapíšeme v tvare:
\(K = \bigcup\limits_{k\in\mathbb{Z}}\{\ {\pi \over 2} + k\pi \}\;.\)
5.
\(1 - \cos 2t = \sin 2t \sin t\)
-
\(1 - \cos^2 t + \sin^2 t = 2 \sin^2 t \cos t\)
(Používame vzorce pre dvojnásobný argument.)
-
\(1 - \cos^2 t + (1 - \cos^2 t) = 2(1 - \cos^2 t)\cos t\)
-
\(2 - 2 \cos^2 t = 2\cos t - 2\cos^3 t\)
-
\(2(1 - \cos^2 t) = 2 (\cos t - \cos^3 t)\)
-
\(\cos^3 t - \cos^2 t - \cos t + 1 = 0\)
-
\(\cos^2 t(\cos t - 1) - 1(\cos t -1) = 0\)
-
\((\cos t - 1)(\cos^2 t - 1) = 0\)
(Využijeme vlastnosť, kedy sa súčin rovná nule (aspoň, keď jeden činiteľ je rovný nule.))
-
\((\cos t - 1)(\cos^2 t - 1) = 0 \Leftrightarrow \cos t - 1 = 0 \vee \cos^2 t - 1 = 0\)
Vyriešime kvadratickú rovnicu (vypočítame diskriminant kvadratickej rovnice a určíme korene rovnice.)
- Prvá možnosť:
\(\cos t - 1 = 0\)
-
\(\cos t_1 = 1\)
-
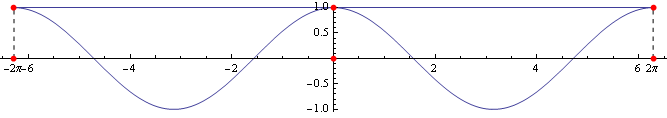
-
\(\cos t_1 = 1 \Rightarrow t_1 = 2k\pi, k \in \mathbb{Z}.\)
- Druhá možnosť:
\(\cos^2 t - 1 = 0\)
-
\(\cos t_2 = \pm 1\)
-
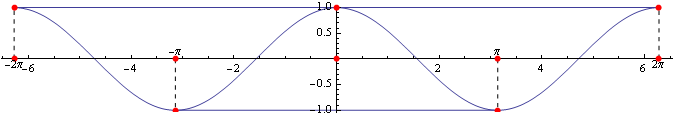
-
\(\cos t_2 = \pm 1 \Rightarrow t_2 = k\pi, k \in \mathbb{Z}.\)
(Zahŕňa v sebe aj prvé riešenie, vplyv periódy.)
- Výsledné riešenie zapíšeme v tvare:
\(K = \bigcup\limits_{k\in\mathbb{Z}}\{\ k\pi \}\;.\)