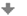\begin{align}
\end{align}
Cvičení - Mocniny s celým mocnitelem
Cvičení 2.10
Cvičení 2.11
Vyber odpovídající výsledek:
Cvičení 2.12
Vypočítej:
a) \(\displaystyle \left(\frac {1} {3} \right)^{-2} + \left(-\, \frac {1} {2} \right)^{-3}
- \left(- \,\frac {1} {3} \right)^{-3} - \left(- \,\frac {1} {5} \right)^{-2} = \;\)
\(\displaystyle 3^2 + (-\,2)^3 - (-\,3)^3 - (-\,5)^2 = \;\)\(\displaystyle 9 - 8 + 27 - 25 = \;\)\(\displaystyle 3\)
b) \(\displaystyle \left(\frac {1} {3} \right)^{-3} + 3^{-1} - \sqrt {3} \left(\sqrt {3} \right)^{-3} = \;\)
\(\displaystyle 3^3 + \frac {1} {3} - \sqrt {3} \cdot \left(\frac {1} {\sqrt {3}}\right)^3 = \;\)\(\displaystyle 27 + \frac {1} {3} - \sqrt {3} \cdot \frac {1} {3 \sqrt {3}} = \;\)\(\displaystyle 27 + \frac {1} {3} - \frac {1} {3} = \;\)\(\displaystyle 27\)
c) \(\displaystyle \left(\sqrt {5} \right)^{-2} + \left(-\, \sqrt {5} \right)^{-2} +
\left(- \,\frac {1} {\sqrt {5}} \right)^{-3} - \left(-\, \frac {1} {\sqrt {5}} \right)^{-3}= \;\)
\(\displaystyle = \left(\sqrt {5}\right)^{-2} + \left(\sqrt {5}\right)^{-2} - \left(\frac {1} {\sqrt {5}} \right)^{-3} + \left(\frac {1} {\sqrt {5}} \right)^{-3}= \;\)\(\displaystyle 2 \cdot \left(\sqrt {5}\right)^{-2} = \;\)\(\displaystyle 2 \cdot \left(\frac {1} {\sqrt {5}}\right)^{2} = \;\)\(\displaystyle \frac {2} {5}\)
d) \(\displaystyle (-\,0,5)^{-2} - (0,5)^{-2} + (0,2)^{-3} + (-\,0,2)^{-3} = \;\)
\(\displaystyle \left(-\, \frac {1} {2} \right)^{-2} - \left(\frac {1} {2} \right)^{-2} +
\left(\frac {1} {5} \right)^{-3} + \left(- \,\frac {1} {5} \right)^{-3} = \;\)
\(\displaystyle = (-\,2)^2 - 2^2 + 5^3 + (-\,5)^3 = \;\)\(\displaystyle 2^2 - 2^2 + 5^3 - 5^3 = \;\)\(\displaystyle 0\)
Cvičení 2.13
Zjednoduš následující výrazy za předpokladu, že
\(a\), \(b\), \(c\), \(d \in \mathbb R - \{0\}\):
a) \(\displaystyle \left(3a^4b^{-6}c^{-4}d^{\,2}\right) \cdot \left(6a^{-2}b^6c^{-3}d^{\,4}\right) = \;\)
\(\displaystyle 18 \cdot a^{\left[4 \, + \, (-2)\right]} \cdot b^{[-6 \, + \,6]} \cdot
c^{\left[-4 \, + \, (-3)\right]} \cdot d^{\,[2 \, + \, 4]} = \;\)\(\displaystyle 18a^2b^0c^{-7}d^{\,6} = \;\)
\(\displaystyle = \frac {18a^2d^{\,6}} {c^7}\)
b) \(\displaystyle \frac {7a^2b^{-4}c^3d^{\,7}} {14a^{-3}b^{-7}c^4d^{\,5}} = \;\)
\(\displaystyle \frac {1} {2} \cdot a^{\left[2 \, - \, (-3)\right]} \cdot
b^{\left[-4 \, - \, (-7)\right]} \cdot c^{[3 \, - \, 4]} \cdot d^{\,[7 \, - \, 5]} = \;\)\(\displaystyle \frac {1} {2} a^5b^3c^{-1}d^{\,2} = \;\)\(\displaystyle \frac {a^5b^3d^{\,2}} {2c}\)
c) \(\displaystyle \frac {15a^{-3}b^6c^{-7}} {2b^7d^{\,-3}} \cdot \frac {8a^2b^{-4}d^{\,2}} {5ac^{-6}} = \;\)
\(\displaystyle 3 \cdot 4 \cdot a^{[-3 \, + \, 2 \, - \, 1]} \cdot b^{[6 \, + \, (-4) \, - \, 7]} \cdot
c^{\left[-7 \, - \, (-6)\right]} \cdot d^{\,\left[2 \, - \, (-3)\right]} = \;\)
\(\displaystyle = 12a^{-2}b^{-5}c^{-1}d^{\,5} = \;\)\(\displaystyle \frac {12d^{\,5}} {a^2b^5c}\)
d) \(\displaystyle \frac {\left(3a^{-2}b^3c^4d^{\,-5} \right)^3} {a^{-4}b^{-3}d^{\,2}} \cdot
\left(\frac {3a^{-3}b^4} {c^{-5}d^{\,2}} \right)^{-2} = \;\)
\(\displaystyle \frac {3^3 \cdot a^{[-2 \, \cdot \, 3]} \cdot b^{[3 \, \cdot \, 3]} \cdot
c^{[4 \, \cdot \, 3]} \cdot d^{\,[-5 \, \cdot \, 3]}} {a^{-4}b^{-3}d^{\,2}}
\cdot \left (\frac {c^{-5}d^{\,2}} {3a^{-3}b^4}\right)^2 = \;\)
\(\displaystyle = \frac {27a^{-6}b^9c^{12}d^{\,-15}} {a^{-4}b^{-3}d^{\,2}} \cdot
\frac {c^{[-5 \, \cdot \, 2]} \cdot d^{\,[2 \, \cdot \, 2]}} {3^2 \cdot a^{[-3 \, \cdot \, 2]} \cdot b^{[4 \, \cdot \, 2]}} = \;\)
\(\displaystyle 27 \cdot a^{\left[-6 \, - \, (-4)\right]} \cdot b^{\left[9 \, - \, (-3)\right]} \cdot
c^{12} \cdot d^{\,[-15 \, - \, 2]} \cdot \frac {c^{-10}d^{\,4}} {9a^{-6}b^{8}} = \;\)
\(\displaystyle = 27a^{-2}b^{12}c^{12}d^{\,-17} \cdot \frac {c^{-10}d^{\,4}} {9a^{-6}b^{8}} = \;\)
\(\displaystyle 3 \cdot a^{\left[-2 \, - \, (-6)\right]} \cdot b^{[12 \, - \, 8]} \cdot
c^{\left[12 \, + \, (-10)\right]} \cdot d^{\,[-17 \, + \, 4]} = \;\)
\(\displaystyle = 3a^{4}b^{4}c^{2}d^{\,-13} = \;\)\(\displaystyle \frac {3a^4b^4c^2} {d^{\,13}}\)
e) \(\left( \displaystyle \frac {2ab^{-3}} {c^{-4}d^{\,2}} \right)^{-2} \div
\left( \displaystyle \frac {a^5c^{-3}} {2b^{-2}d^{\,2}} \right)^{-3} = \;\)
\(\displaystyle \left(\frac {c^{-4}d^{\,2}} {2ab^{-3}}\right)^{2} \cdot
\left(\frac {a^5c^{-3}} {2b^{-2}d^{\,2}}\right)^3 = \;\)
\(\displaystyle = \frac {c^{[-4 \, \cdot \, 2]} \cdot d^{\,[2 \, \cdot \, 2]}} {2^2 \cdot a^{[1 \, \cdot \, 2]}
\cdot b^{[-3 \, \cdot \, 2]}} \cdot
\frac {a^{[5 \, \cdot \, 3]} \cdot c^{[-3 \, \cdot \, 3]}} {2^3 \cdot b^{[-2 \, \cdot \, 3]} \cdot d^{\,[2 \, \cdot \, 3]}} = \;\)
\(\displaystyle \frac {c^{-8}d^{\,4}} {4a^2b^{-6}} \cdot \frac {a^{15}c^{-9}} {8b^{-6}d^{\,6}} = \;\)
\(\displaystyle \frac {1} {32} \cdot \frac {a^{[15 \, - \, 2]} \cdot c^{\left[-8 \, + \, (-9)\right]}
\cdot d^{\,[4 \, - \,6]}} {b^{\left[-6 \, + \, (-6)\right]}} =\;\)
\(\displaystyle = \frac {1} {32} \cdot\frac {a^{13}c^{-17}d^{\,-2}} {b^{-12}} = \;\)
\(\displaystyle \frac {1} {32} \cdot\frac {a^{13}b^{12}} {c^{17}d^{\,2}}\)
f) \(\displaystyle \frac {\left(b^{-4}d^2 \right)^{-3}} {5a^{-6}b^2c^4} \div
\left(\frac {a^2bc^5} {5a^3b^{-4}d^{\,6}} \right)^2 = \;\)
\(\displaystyle \frac {b^{\left[-4 \, \cdot \, (-3)\right]} \cdot
d^{\,\left[2 \, \cdot \, (-3)\right]}} {5a^{-6}b^2c^4} \cdot
\frac {5^2 \cdot a^{[3 \, \cdot \, 2]} \cdot b^{[-4 \, \cdot \, 2]} \cdot d^{\,[6 \, \cdot \, 2]}} {a^{[2 \, \cdot \, 2]} \cdot b^{[1 \, \cdot \, 2]} \cdot c^{[5 \, \cdot \, 2]}} = \;\)
\(\displaystyle = \frac {b^{12}d^{\,-6}} {5a^{-6}b^2c^4} \cdot \frac {25a^6b^{-8}d^{\,12}} {a^4b^2c^{10}} = \;\)
\(\displaystyle \frac {5a^{\left[6 \, - \, (-6 \, + \, 4)\right]}\cdot
b^{\left[12 \, + \, (-8) \, - \, (2 \, + \, 2)\right]} \cdot d^{\,[-6 \, + \, 12]}}
{c^{[4 \, + \, 10]}} = \;\)\(\displaystyle \frac {5a^8d^{\,6}} {c^{14}}\)
Cvičení 2.14
Vyber odpovídající zápis:
Cvičení 2.15
Přepiš následující údaje tak, aby jejich číselná hodnota byla
ve tvaru \(a \cdot 10^n\),
kde \(1 \leq a \leq 10\), \(n \in \mathbb Z\):
a) Světlo se pohybuje ve vakuu rychlostí \(v = 300\,000\) km/s.
 \(\; \; \; \; v = 3 \cdot 10^5\) km/s.
\(\; \; \; \; v = 3 \cdot 10^5\) km/s.
b) Obvod \(o\) rovníku je přibližně \(6\,371\,000\) m.
 \(\; \; \; \; o = 6,371 \cdot 10^6\) m.
\(\; \; \; \; o = 6,371 \cdot 10^6\) m.
c) Průměr \(d\) červené krvinky se pohybuje kolem \(0,000\,007\,2\) m.
 \(\; \; \; \; d = 7,2 \cdot 10^{-6}\) m.
\(\; \; \; \; d = 7,2 \cdot 10^{-6}\) m.
Cvičení 2.16
Daná čísla nejdříve zapiš ve tvaru \(a \cdot 10^n\),
kde \(1 \leq a \leq 10\), \(n \in \mathbb Z\), a poté zjednoduš:
a) \(\displaystyle \frac {0,000\,45} {3\,000} \cdot \frac {20\,000} {0,01} = \;\)
\(\displaystyle \frac {4,5 \cdot 10^{-4}} {3 \cdot 10^3} \cdot \frac {2 \cdot 10^4} {1 \cdot 10^{-2}} = \;\)\(\displaystyle \frac {9 \cdot 10^0} {3 \cdot 10^1} = \;\)\(\displaystyle 3 \cdot 10^{-1} = \;\)\(\displaystyle 0,3\)
b) \(\displaystyle \frac {0,5} {1\,500\,000} \cdot \frac {240\,000} {0,004} = \;\)
\(\displaystyle \frac {5 \cdot 10^{-1}} {1,5 \cdot 10^6} \cdot \frac {2,4 \cdot 10^5} {4 \cdot 10^{-3}} = \;\)\(\displaystyle \frac {12 \cdot 10^4} {6 \cdot 10^3} = \;\)\(\displaystyle 2 \cdot 10^1 = \;\)\(\displaystyle 20\)
c) \(\displaystyle \frac {25\,000} {10} \div \frac {0,000\,005} {0,000\,8} = \;\)
\(\displaystyle \frac {2,5 \cdot 10^4} {1 \cdot 10^1} \cdot \frac {8 \cdot 10^{-4}} {5 \cdot 10^{-6}} = \;\)\(\displaystyle \frac {20 \cdot 10^0} {5 \cdot 10^{-5}} = \;\)\(\displaystyle 4 \cdot 10^5 = \;\)\(\displaystyle 400\,000\)
d) \(\displaystyle \frac {3\,000\,000} {20\,000} \div \frac {0,000\,015} {0,005\,3} = \;\)
\(\displaystyle \frac {3 \cdot 10^6} {2 \cdot 10^4} \cdot \frac {5,3 \cdot 10^{-3}} {1,5 \cdot 10^{-5}} = \;\)\(\displaystyle \frac {15,9 \cdot 10^3} {3 \cdot 10^{-1}} = \;\)\(\displaystyle 5,3 \cdot 10^4 = \;\)\(\displaystyle 53\,000\)
Cvičení 2.17 
Zjedoduš za předpokladu, že \(x\), \(y\),
\(z \in \mathbb R - \{0\}\) a
\(a\), \(b\), \(c \in \mathbb Z\):
a) \(\large \left(x^{[a \, - \, b]} \cdot y^{[2a \, +\, 2b]}\right) \cdot \left(x^b \cdot y^{-2b} \right) = \;\)
\(\large x^a \cdot y^{2a} = \;\)\(\large \left(x \cdot y^2\right)^a\)
b) \(\LARGE \frac {\left(x^{-b} \cdot y^{2b}\right)^c} {\left(x^a \cdot z^3\right)^b} \cdot \frac {\left(x^b \cdot z\right)^a} {\left(x^{3c} \cdot y^{-4c}\right)^b} = \;\)
\(\LARGE \frac {x^{-bc} \cdot y^{2bc}} {x^{ab} \cdot z^{3b}} \cdot \frac {x^{ab} \cdot z^a} {x^{3bc} \cdot y^{-4bc}} = \;\)\(\LARGE \frac {y^{6bc} \cdot z^{[a \,-\, 3b]}} {x^{4bc}}\)
c) \(\LARGE \frac {x^{2a} \cdot z^{-b}} {y^{[4a \,-\, 1]}} \large \div \LARGE \frac {z^{[2 \,-\, b]} \cdot x^{[a \,+\, b]}} {y^{[2b \,-\, 3]}} = \;\)
\(\LARGE \frac {x^{2a} \cdot z^{-b}} {y^{[4a \,-\, 1]}} \cdot \frac {y^{[2b \,-\, 3]}} {z^{[2 \,-\, b]} \cdot x^{[a \,+\, b]}} = \;\)\(\LARGE \frac {x^{[a \,-\, b]}} {y^{[4a \,-\, 2b \,+\, 2]} \cdot z^2}\)
d) \(\LARGE \left[ \frac {\left(x^{2a} \cdot y^3\right)^{-c}x} {z^c} \right]^{-b} \cdot \left(\frac {z^{4c}} {x^{-1} \cdot y^{2c}}\right)^b = \;\)
\(\LARGE \frac {x^{2abc} \cdot y^{3bc} \cdot x^{-b}} {z^{-bc}} \cdot \frac {z^{4bc}} {x^{-b} \cdot y^{2bc}} = \;\)\(\large x^{2abc} \cdot y^{bc} \cdot z^{5bc} = \;\)
\(\large = \left(x^{2a} \cdot y \cdot z^5\right)^{bc}\)
Cvičení 2.18 
Vypočítej:
a) \(\displaystyle \frac {(-\,5)^{-2} \cdot \left(- \,\frac {1} {5} \right)^{-3} + \left(\frac {1} {2}\right)^{-2} \cdot 2^{-3} \cdot \left(- \, \frac {1}{6}\right)^{-2}}
{\left(\frac {1} {27} \right)^0} = \;\)
\(\displaystyle \frac {\frac {1} {25} \cdot (-\,125) + 4 \cdot \frac {1} {8} \cdot 36} {1} = \;\)
\(\displaystyle = -\,5 + \frac {1} {2} \cdot 36 = \;\)\(\displaystyle -\,5 + 18 = \;\)\(\displaystyle 13\)
b) \(\displaystyle \frac {2^{-2} \cdot \left(- \, \frac {1} {2} \right)^{-4} + \left( \frac {1} {5} \right)^{-1} \cdot \left( \frac {1} {3} \right)^{-4} \cdot 3^{-3} - \left(- \, \frac {1} {5} \right)^0}
{2 \cdot (-3)^{-2} \cdot \left(- \, \frac {1} {3}\right)^{-4} - \left(- \, \frac {1} {3}\right)^{-2}} = \;\)
\(\displaystyle \frac {\frac {1} {4} \cdot 16 + 5 \cdot 81 \cdot \frac {1} {27} - 1} {2 \cdot \frac {1} {9} \cdot 81 - 9} = \;\)
\(\displaystyle = \frac {4 + 5 \cdot 3 - 1} {2 \cdot 9 - 9} =\)\(\displaystyle \frac {4 + 15 - 1} {18 - 9} = \;\)\(\displaystyle \frac {18} {9} = \;\)\(\displaystyle 2\)