



Next: New examples 2
Up: New examples
Previous: Semicircle Continuum
Boxes Continuum is a nice continuum.
Let
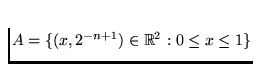 ;
;
for each
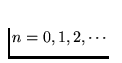 and each
and each
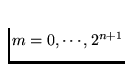 ,
let
,
let
finally, let
1) X is an example of an non-hlc continuum which
is not regular.
2) X is the union of two regular continua.
See Nadler 10.38, p.186.
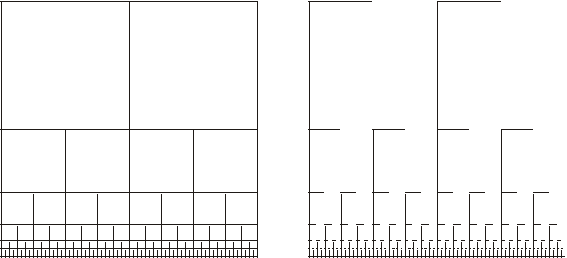
Figure (
A
)
Union of two dendrites, a non-hlc
Boxes Continuum
Source files:
a.eps .
a.gif .
a.mws .
a.txt .
latex.tex .
title.txt .
Here you can
read Notes or
write to Notes.
Janusz J. Charatonik, Pawel Krupski and Pavel Pyrih
2001-02-21
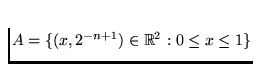 ;
;
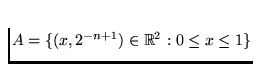 ;
;
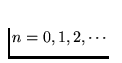 and each
and each
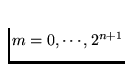 ,
let
,
let
![\begin{displaymath}
X = \left [\bigcup_{n=1}^\infty A_n\right ]
\cup
\left [\big...
...infty \left ( \bigcup_{m=0}^{2^{n+1}} B_{n,m}\right
)\right ].
\end{displaymath}](img105.gif)
