 |
|
|
Sokolovská 83 186 75 Praha 8 - Karlín Czech Republic [+420] 9 5155 3362 kucera@karlin.mff.cuni.cz |
|---|
Research

Main scientific results
- Robust numerical scheme for low Mach number (10-6) as well as transonic and supersonic compressible flows [2007].
- Robust numerical scheme for fluid structure interactions using the ALE method [2010].
- Optimal error estimates for DG applied to a nonlinear convection and nonlinear diffusion problem [2010].
- Analysis of a space-time DG method for nonlinear convection-diffusion [2011].
- Simulation of voice formation in human vocal chords [2013].
- Error estimates uniform w.r.t. diffusion for DG for nonlinear convection-diffusion and purely convective problems [2014].
- "Nice" triangles cannot be subdivided into "ugly" triangles only. [2015].
- The circumradius condition (estimate) is a simple scaling of the standard maximum angle condition [2016].
- Development of a numerical method for cell segmentation based on the level set method [2016].
- Efficient numerical scheme for a nonlocal hydrodynamic model of flocking dynamics [2018].
- Generalization of known sufficient conditions for FEM convergence [preprint].
- Formulation of a necessary condition for FEM convergence, construction of various counterexamples [preprint].
- Error estimates uniform w.r.t. time for DG for advection-diffusion problems [2019].
- Proof of consistency with the low Mach incompressible limit for a general class of linearized schemes for the compressible Euler equations [2022].
- "Nice" tetrahedra cannot be subdivided into "ugly" tetrahedra only [2022].
- Generalization of Godunov's numerical flux to network junctions [2023].
- Making finite elements work on degenerate elements [2024].
Ongoing work
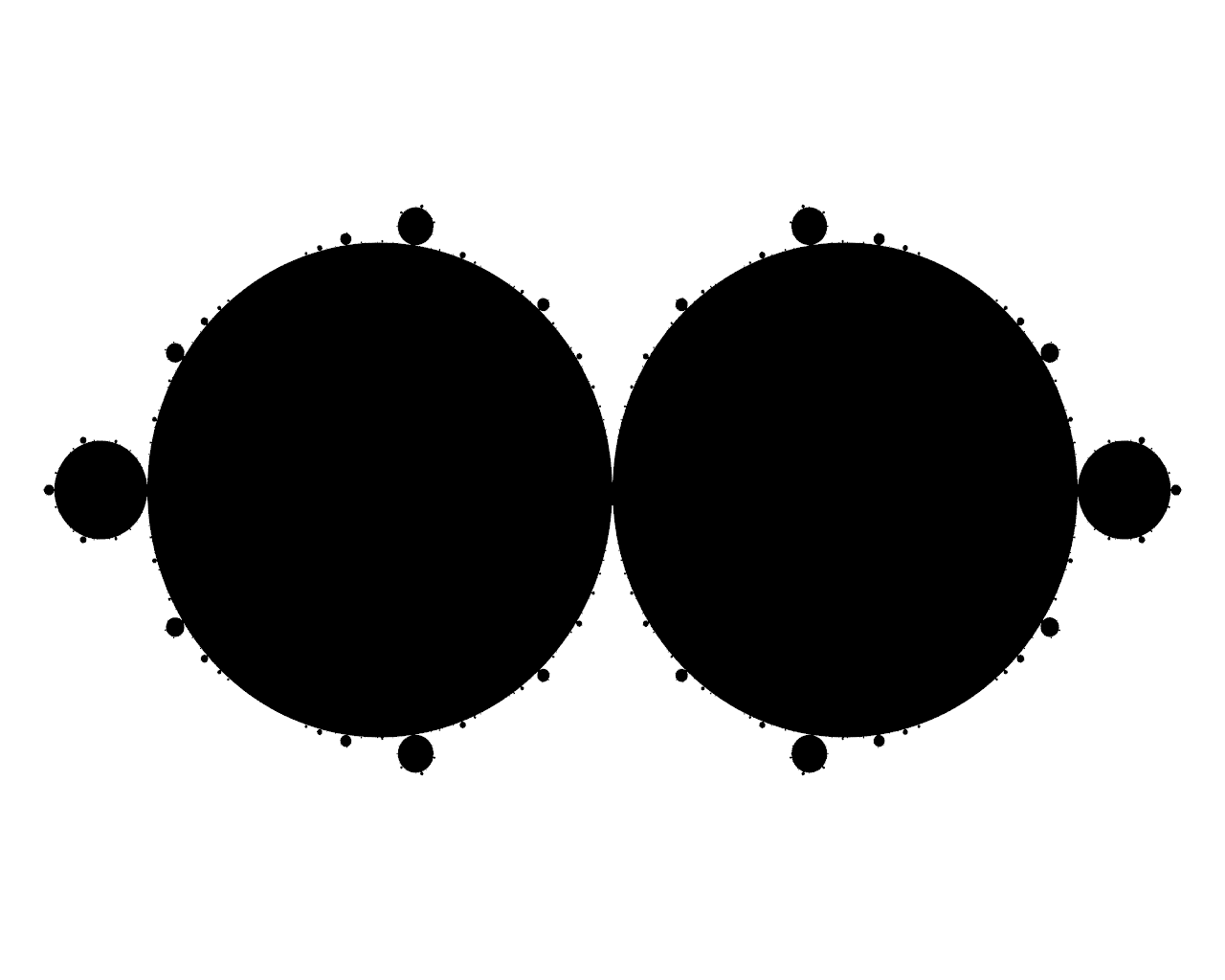
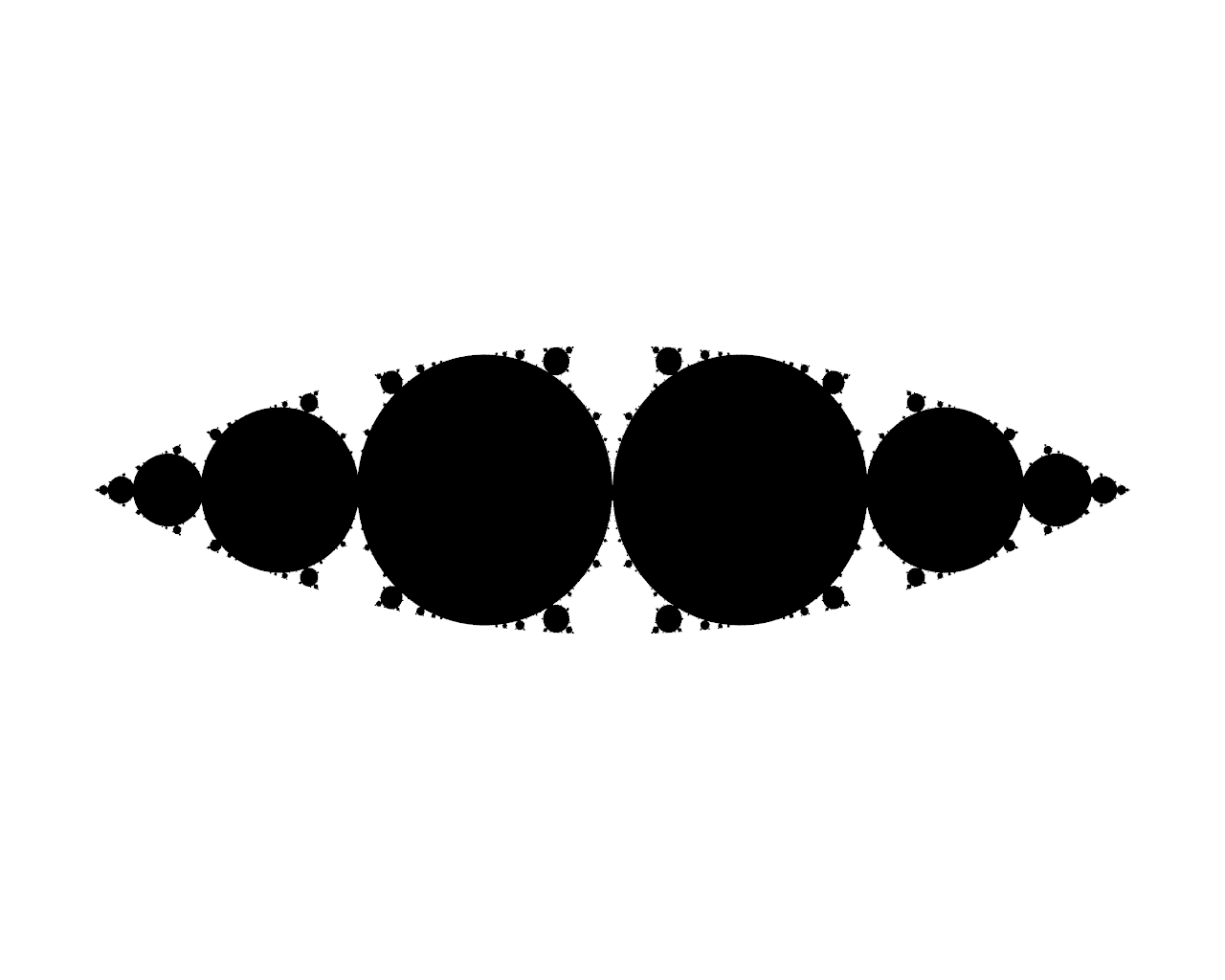
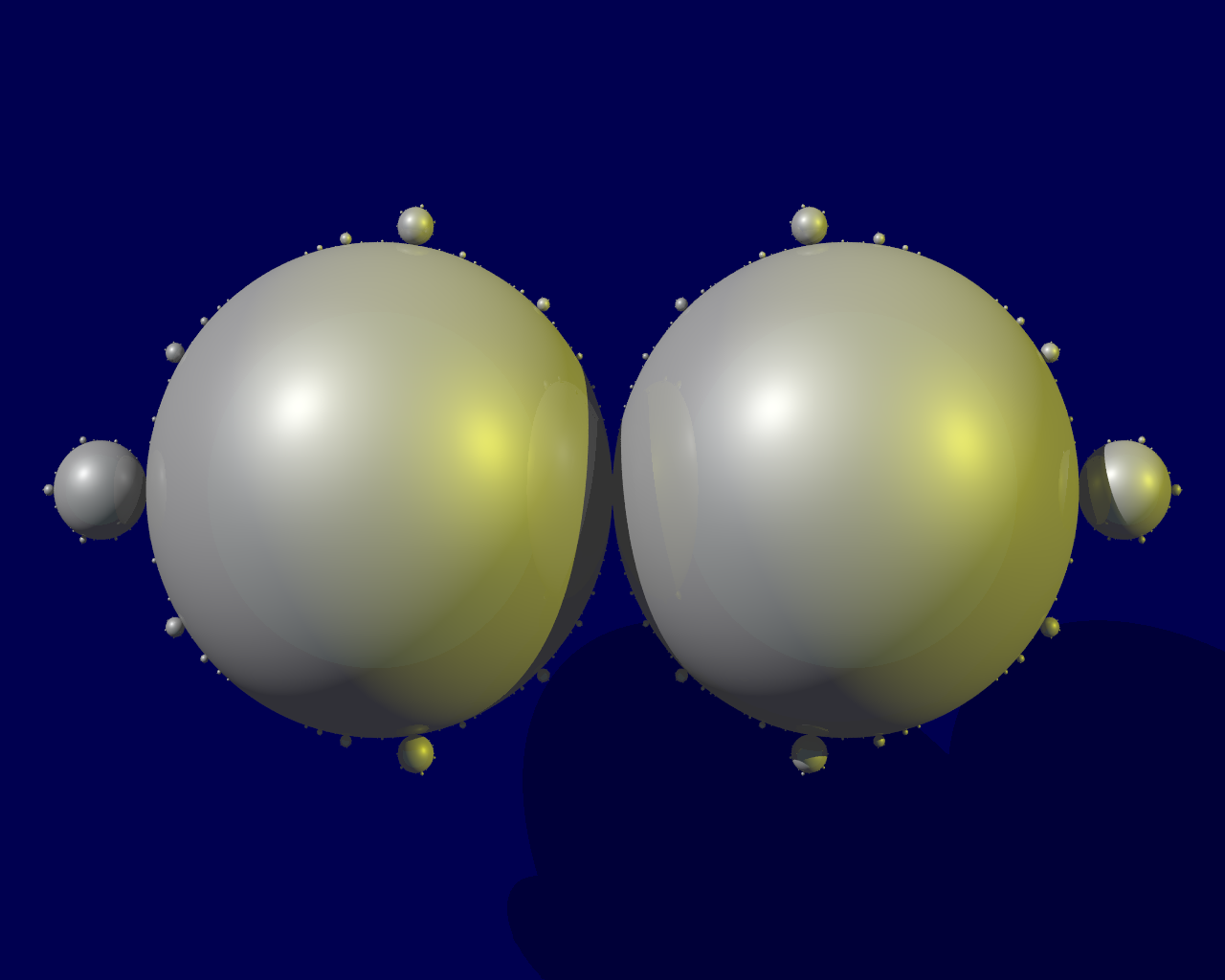
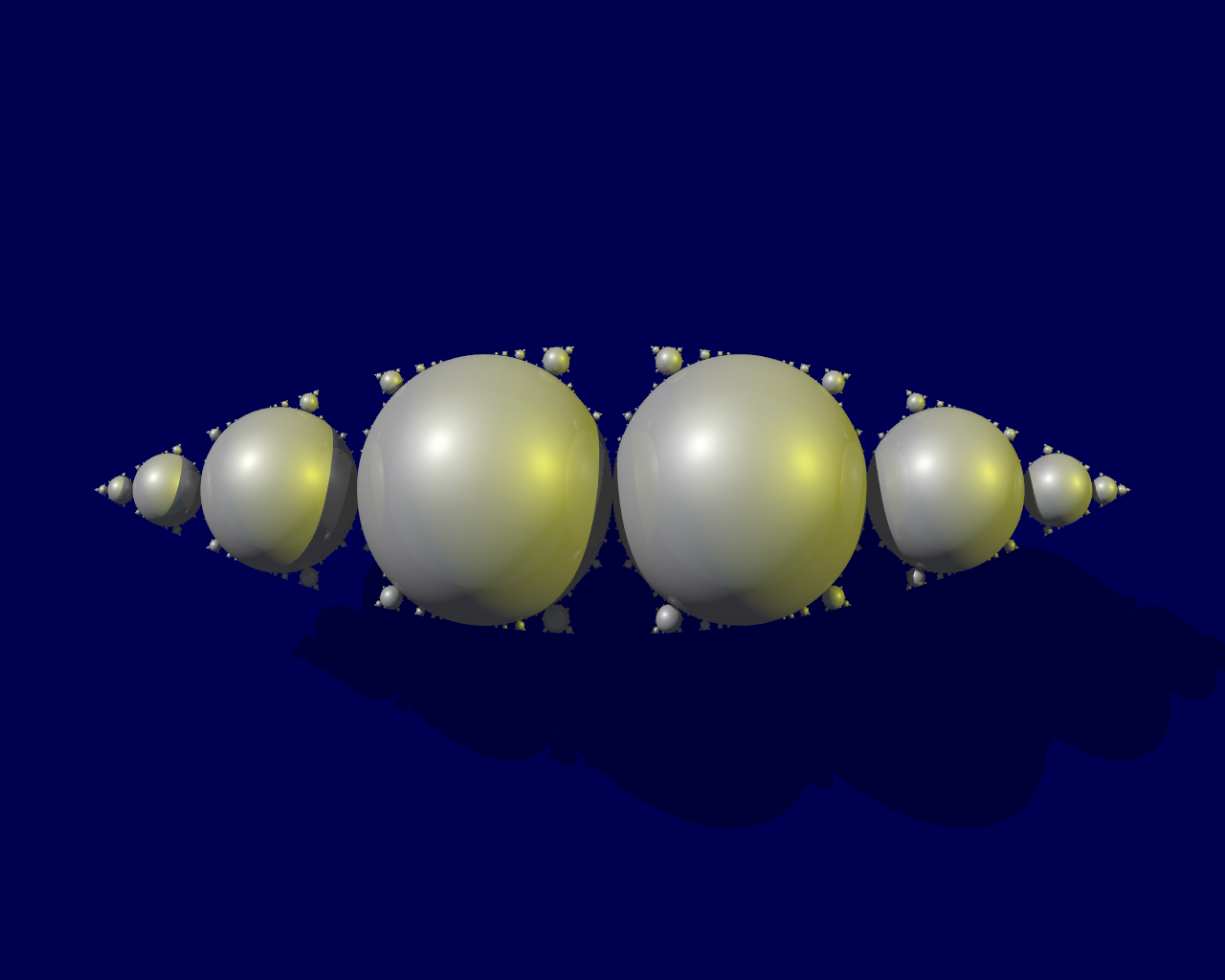
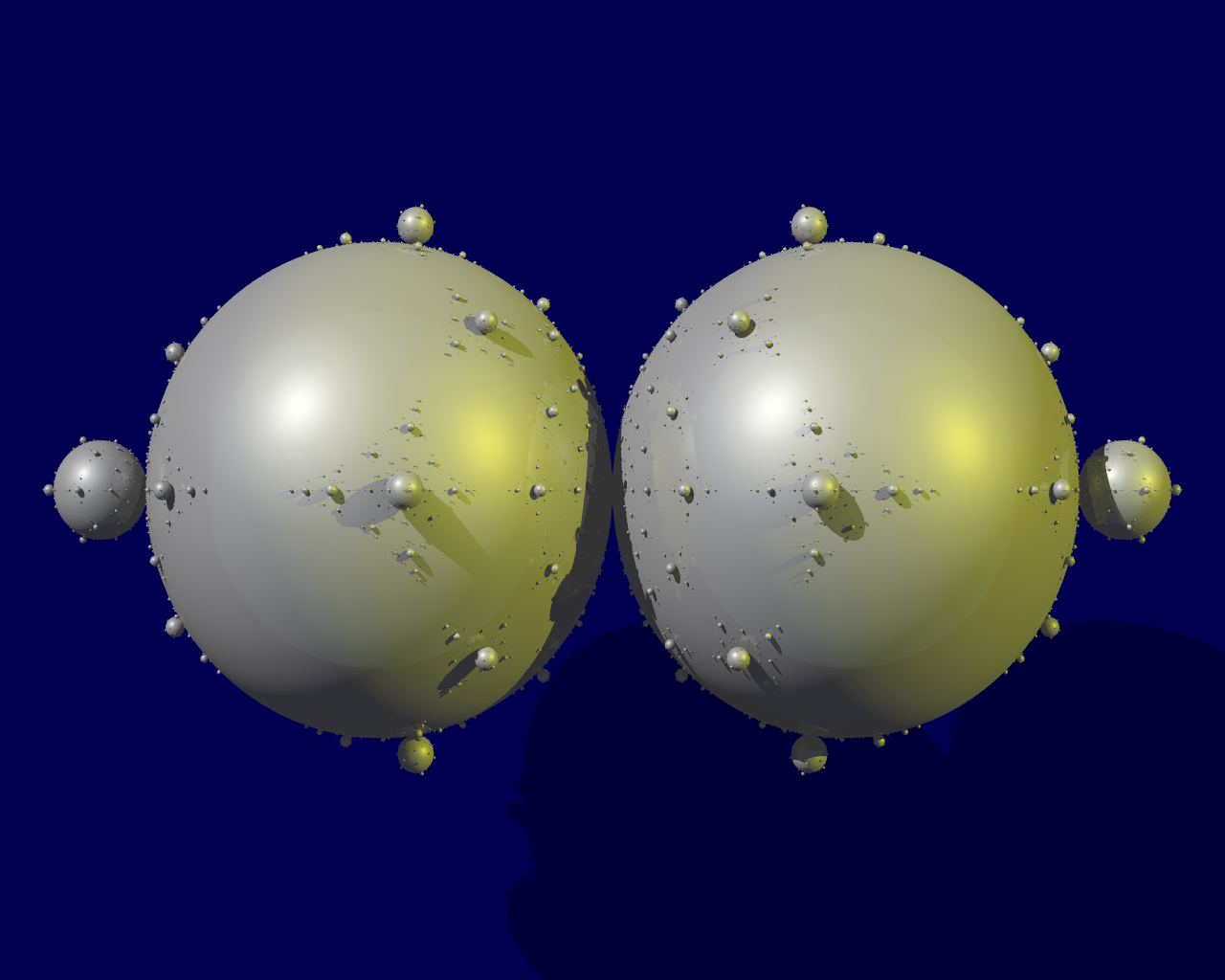
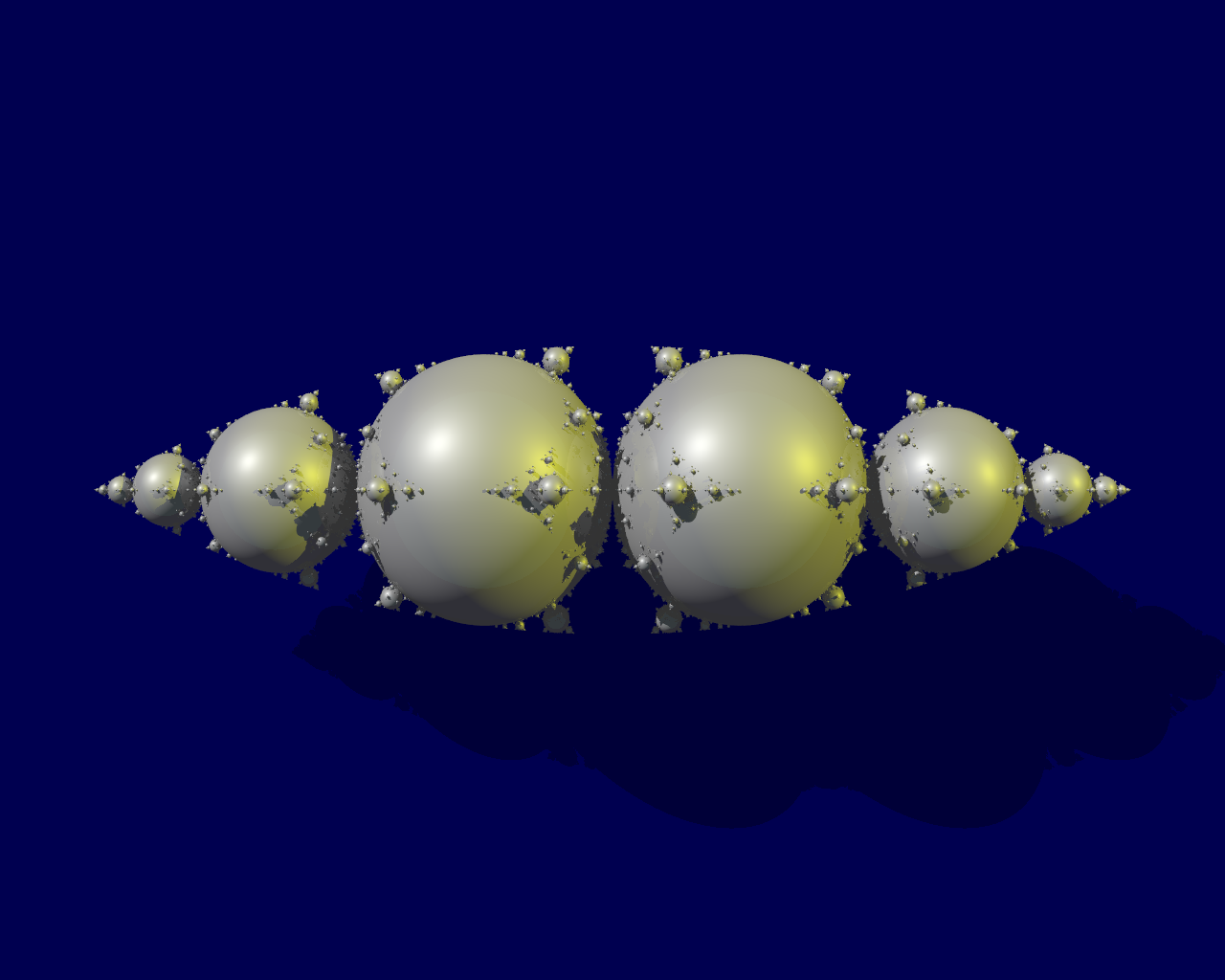
Currently I am digressing from my usual field of numerical analysis
into the world of 3D fractals and chaotic dynamical systems. This was
always a hobby of mine and now thanks to the Neuron fund, I can
finally start doing serious research on this topic. As a preview, here are some
POV-Ray pictures of 2D Kleinian limit sets, their trivial extensions into 3D
and nontrivial 3D extensions.
 |
 |
 |
 |
 |
 |