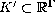 such that
such that
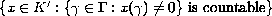 is dense in K'.
is dense in K'.
Definition. A compact space K
is called Valdivia if it is homeomorphic to some
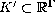 such that
such that
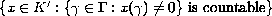 is dense in K'.
is dense in K'.
PROBLEM 1 (2 beers)
Is there a scattered Valdivia compactum containing a copy of
![$[0,\omega_2]$](obr/0om2.gif) ?
?
A topological space is scattered if any its nonempty subset has a relatively isolated point.
PROBLEM 2 (Asked at 29th Winter School in Lhota nad Rohanovem,
February 2001) Let ![$X=\ell_1([0,\pi/2])$](obr/Xjel10pi2.gif) and
and ![$Y=\{x\in X: \sum\limits_{t\in[0,\pi/2]}x(t)\cos t=0\ \& \ \sum\limits_{t\in[0,\pi/2]}x(t)\sin t=0\}$](obr/Yanihsincos.gif) .
Is the dual unit ball of Y, in its weak* topology,
a Valdivia compactum?
.
Is the dual unit ball of Y, in its weak* topology,
a Valdivia compactum?
Possible answers:
a) No. (3 beers)
b) Yes, and the homeomorphism in the definition of a Valdivia compactum
may be chosen linear. (2 beers)
c) Yes, but the homeomorphism cannot be chosen linear. (10 beers)
PROBLEM 3 (3 beers) Let K and L
be nonempty compact spaces such that
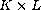 is Valdivia.
Are K and L Valdivia, too?
is Valdivia.
Are K and L Valdivia, too?
Remark: Yes, if each one of them has at least one
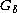 -point (easy) or if at least one
of them has a dense set of
-point (easy) or if at least one
of them has a dense set of 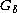 -points
(not easy).
-points
(not easy).